THE PV PRESSURE ↔ FLUID VELOCITY SWAP
 Will it go round in circles?
Will it go round in circles?
Will it fly high like a bird up in the sky?
("Will It Go Round in Circles," Billy Preston & B. Fisher, 1973)
THE PV PRESSURE SWAP WITH FLUID VELOCITY
PTOA Readers and Students who are reading the PTOA Segments in the intended sequential order just learned in PTOA Segment #158 that the PV Flowrate is totally dependent upon a Pressure Differential (aka ΔP) to exist.
There is a second crucial relationship between the PV Pressure and the Velocity component of the PV Flowrate:
An increase in the Velocity of a flowing fluid will result in a decrease of the fluid's PV Pressure and then ...
The recovery and increase of the PV Pressure in the flowing fluid will result in a corresponding decrease of its Velocity.
 Oh no.
Oh no.
Fred's a little confused and getting jittery.
But he won't be after finishing this PTOA Segment #159 which is dedicated to defining and understanding
The PV Pressure ↔ Fluid Velocity Swap!
THE PV (VOLUMETRIC) FLOWRATE HAS TWO COMPONENTS:
VELOCITY AND (FLOW THROUGH) AREA
 By now, PTOA Readers and Students could create a Power Point presentation in their sleep that explains the dependent relationship between the PV Flowrate and ΔP.
By now, PTOA Readers and Students could create a Power Point presentation in their sleep that explains the dependent relationship between the PV Flowrate and ΔP.
A quality presentation would not fail to mention that the Universe demands that this dependent relationship apply to ALL flowing fluids, not just those confined to flow within the pipes of a processing facility.
Heck ...
It's easier to observe the PV Pressure - PV (Volumetric) Flowrate relationship in nature because nobody has x-ray vision to see what's going on in all those pipes!

The Ohio River (right side) joins the Mississippi River (left side) and the combined river flows "downward" to the area of less pressure ... the Gulf of Mexico.
For example ...
All rivers also flow from an area of HIGH PRESSURE to an area of LOW PRESSURE.
In fact,
a "river" that is not flowing because of loss of ΔP is not a river at all but rather what we Earthlings recognize as the pooled water of "a lake!"

The "Flowrate" of a river is what we Earthlings call "a current."
Yet the current of a river is none other than how fast the water is flowing ... aka its Velocity.
 The river's Velocity ..."V" ... is one of the two components that comprise its (Volumetric) Flowrate, "Q."
The river's Velocity ..."V" ... is one of the two components that comprise its (Volumetric) Flowrate, "Q."

The PV (Volumetric) Flowrate is composed of the Fluid's Velocity (V) multiplied by the (Flow Through) Area (A) of whatever the fluid is flowing through.
The other component of "Q" is (Flow Through) Area, "A."
Multiplying the Velocity and (Flow Through) Area of any flowing fluid results in quantifying its Volumetric Flowrate "Q" in units of Volume/time ...
like "standard cubic feet per hour" (scft3/hr) or "cubic meters per hour" (M3/hr) which could be converted into English unit equivalents of, say, "barrels per day" (Bbls/day) or "gallons per hour" (gal/hr).
The units are consistent on both sides of the expression that defines "Q" because:
 Velocity is measured in units of length per unit time ...
Velocity is measured in units of length per unit time ...
For example "feet/min" or "feet/sec" or "meters/minute" or "meters/sec."
 And (Flow Through) Area is exactly what it says it is ...
And (Flow Through) Area is exactly what it says it is ...
the cross-sectional area that the fluid is flowing through! Like, Duh!
As you would expect ...
the (Flow Through) Area is measured in units of area ... like "ft2" or "m2."
It's a little tricky to calculate the exact (Flow Through) Area for riverbeds because they are basically partially filled ducts ... and the geological features below the surface of the water must be taken into account because they impact the total (Flow Through) Area.
Fortunately a study of current flow (aka river Velocity) can help reveal what lies beneath the river surface that obstructs flow!
A STUDY OF A RIVER'S FLUID VELOCITY
The Santa Clara River graphic below colorfully depicts how the Velocity of the Santa Clara River changes as it flows through several of its bends.
- Yellow is a Velocity measured to be 2-4 feet/sec; PTOA Readers and Students should notice how this low Velocity occurs near the river bank. The color yellow does not ever appear in the center of the flowing river.

- Lightest Blue appears just off the bank and indicates the river Velocity measured at 6-8 feet/s.
- Aqua Blue appears in the center of the river and indicates a substantially faster Velocity of 12-15 feet/s.
- Dark Blue patches of color indicate the fastest river Velocity of 18-21 feet/s.

Aha!
The dark blue color that indicates the fastest river Velocity always occurs where the river has a "pinch point" ... a place where the width of the river narrows.
Why does the Velocity of the flowing river increase at a pinch point?

The same confluence of the Ohio and Mississippi Rivers as shown above ... only this picture was taken during the flood of 2011.
If the river Velocity did not increase at a pinch point, then the flowing water would "bunch up" and flood the surrounding area.
The high dollar instructional jargon word to use here is "continuity" as in:
To maintain the overall flow continuity of the river, the river's Velocity changes with the width of the river;
The river's Velocity increases when the width of the river narrows and decreases when the width of the river widens.
THE OBSERVATIONS OF A FLOWING RIVER DIRECTLY APPLY TO A FLUID THAT FLOWS THROUGH A RIGID PIPE
 PTOA Readers and Students should not be at all surprised to learn that the Velocity behavior observed with the flowing Santa Clara River is the same Velocity behavior that would be observed in the fluids that flow through all the processing pipes ... if we just had the x-ray vision to look inside the mess of pipes that distribute all those fluids from one processing point to the other!
PTOA Readers and Students should not be at all surprised to learn that the Velocity behavior observed with the flowing Santa Clara River is the same Velocity behavior that would be observed in the fluids that flow through all the processing pipes ... if we just had the x-ray vision to look inside the mess of pipes that distribute all those fluids from one processing point to the other!
There is one noteworthy difference between the two case studies:
The (Flow Through) Area of a rigid metal or plastic pipe is constant and determined from the interior pipe radius.
 So the (Flow Through) Area of any pipe is easy to calculate because it is simply the area of the circle with the interior diameter (and hence radius) of the pipe:
So the (Flow Through) Area of any pipe is easy to calculate because it is simply the area of the circle with the interior diameter (and hence radius) of the pipe:
(Flow Through) Area = π * (Internal Radius of Pipe) 2
Since the (Flow Through) Area is unchangeable, only the Velocity component of the PV (Volumetric) Flowrate "Q" can change in a fluid-filled pipe.
So the "Velocity Profile" that the flowing fluid develops is caused by only the fluid's Velocity and looks like the schematic below:

Brilliant PTOA Readers and Students will notice these similarities between the above Velocity Profile graphic and the map of the Santa Clara River current:
- The slowest fluid Velocity is the fluid that is closest to the interior pipe wall. The fluid closest to the pipe wall cannot flow as fast as the rest of the fluid because it is held back by interacting with the interior wall's surface (aka "friction").
- The fastest fluid Velocity is at the center of the pipe.
- Thus there is a "Velocity Profile" that increases radially and gradually from the slowest Velocity "layer" observed at the pipe wall to the fastest Velocity "layer" that flows at the center of the pipe.
If we were to apply color mapping to define the fluid Velocities shown in the above rigid pipe graphic the result would be something like this:
 The red flow at the center would have the fastest Velocity.
The red flow at the center would have the fastest Velocity.
The yellow flow that radially surrounds the red area would have the next fastest Velocity.
The green area that radially surrounds the yellow area would have a slower Velocity than the yellow Velocity area ... but a greater Velocity than ...
The light blue Velocity area which radially surrounds the green area. This radial area has the slowest fluid Velocity area because it is hung up by friction while interacting with the dark blue interior wall.
 Take another look at the flow gushing from the pipe in the nearby photo.
Take another look at the flow gushing from the pipe in the nearby photo.
Who can discern the "Velocity Profile?"
The trained eye can discern the significantly greater Velocity of the water that protrudes outward from the center of the pipe as compared to the Velocity of the water that is hindered by friction at the wall of the pipe.
If that "Velocity Profile" was too hard to see ... can you discern the "Velocity Profile" of the fluid flowing through the laboratory-study glass pipe below?

The "Velocity Profile" can be seen fully developed on the far right side of the photo; its cone shape looks remarkably like the graphic below: Congratulations!
Congratulations!
All PTOA Readers and Students just learned how to identify what is called "Laminar Flow" and now know that it can be identified by:
- Radial "layers" of flowing fluid velocities which make a ...
- "Velocity Profile" that is slowest at the wall and gradually increases radially "in layers" to become fastest at the center of the pipe where it forms a ...
- Cone shape.
Now ... let's throw a monkey wrench into this perfect Laminar Flow "Velocity Profile" by changing the diameter of the pipe that the fluid must flow through!

This is a Venturi Meter. It is used to measure the Flowrate of a gas.
TA-DA! THE PV PRESSURE ↔ FLUID VELOCITY SWAP
 Which brilliant PTOA Readers and Students wish to predict what will happen to the Velocity of a flowing fluid when the diameter of the rigid pipe is swaged down into a tighter throat?
Which brilliant PTOA Readers and Students wish to predict what will happen to the Velocity of a flowing fluid when the diameter of the rigid pipe is swaged down into a tighter throat?
Hint! Remember the concept of "continuity" ... the fluid is not allowed to "bunch up" when the (Flow Through) Area is reduced.
Who thinks the fluid Velocity will increase as it did in the Santa Clara River?
If you said YES INDEEDO then you are CORRECTAMUNDO!
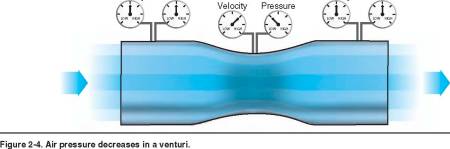
The above graphic illustrates how the fluid's Velocity and the PV Pressure swap in magnitude as they pass through an area of restriction.
The graphic shows:
- Three PIs can measure and indicate the PV Pressure of the flowing fluid before, during, and after flowing through the area of restriction (aka smaller diameter pipe).
- Three "Velocity meters" are located to the left of each PI and measure the corresponding fluid Velocity before, during, and after flowing through the area of restriction.
The PV Pressure indicators and Velocity meters tell the following tale:
While passing through the restriction, the fluid's Velocity increases temporarily while the PV Pressure decreases.
Once further "downstream" from the restriction, the fluid's PV Pressure increases and recovers to where it was originally as the fluid's Velocity decreases to where it had been originally.

The decreased diameter of the pipe INCREASES FLUID VELOCITY and DECREASES FLUID PRESSURE. The former Fluid Velocity and Pressure are restored further "downstream" from the "throat."
This swapping of the PV Pressure for an increase in fluid Velocity ... and back again is called
THE PV PRESSURE ↔ FLUID VELOCITY SWAP
 Got it, Fred?
Got it, Fred?
THE POWER OF THE SWAP
Mankind has learned how to harness the power of the PV Pressure ↔ Fluid Velocity Swap to create amazing process technologies.
 The PV Pressure ↔ Fluid Velocity Swap is used to measure the PV Flowrate of gases or liquids ... as long as the fluids meet the criteria that characterizes "Laminar flow."
The PV Pressure ↔ Fluid Velocity Swap is used to measure the PV Flowrate of gases or liquids ... as long as the fluids meet the criteria that characterizes "Laminar flow."

The difference between high pressure h1 and low pressure h2 is a head measurement that can be converted into a Flowrate measurement. Nifty!
In the future PTOA PV Flowrate Focus Study Area, PTOA Readers and Students who desire to know more about automatic instrumentation ...

PTOA Readers & Students learned how the PV(Volumetric) Flowrate is related to a head pressure in PTOA Segment #158.
will learn how an intentionally placed restriction in the path of a fluid that meets the criteria of Laminar flow ....

Three types of flow obstructions intentionally placed to create a head pressure measurement that can be converted into a PV Flowrate measurement.
can utilize the pressure-change to generate a head measurement which can thence be converted into a PV Flowrate measurement!
My, my that's pretty nifty!
Another nifty use of the PV Pressure ↔ Fluid Velocity Swap is adding Pressure to liquids.
In the relatively recent PTOA Segment #153, PTOA Readers and Students learned that gases are compressible but liquids are not.
 Since compression won't work to increase the PV Pressure of a liquid, Mankind has ingeniously harnessed the power of the PV Pressure ↔ Fluid Velocity Swap to build up the PV Pressure of a liquid by decreasing the liquid's Velocity.
Since compression won't work to increase the PV Pressure of a liquid, Mankind has ingeniously harnessed the power of the PV Pressure ↔ Fluid Velocity Swap to build up the PV Pressure of a liquid by decreasing the liquid's Velocity.
Centrifugal Pumps will be featured soon in this PTOA PV Pressure Focus Study Area. Stay tuned!
ANSWER TO DIY IN PTOA SEGMENT #158:
 Is there flow going through this pipe?
Is there flow going through this pipe?
The pipe is pressurized with a fluid but is currently blocked in from any flow. We can conclude that there is no flow ongoing through the pipe because there is no pressure drop in the line. No pressure drop, no flow!
If so, in what direction is the flow going?
Since there is no flow through the pipe, there is no direction to the flow.
When flow commences in the future, the flow will be from the source of higher pressure ...
For example, if the fluid in the pipe is a liquid ...
The source of high pressure might be from the head pressure provided by a liquid filled tank or from the discharge of a pump.
If the fluid in the pipe is a gas, the source of high pressure might be from the discharge of a compressor.
TAKE HOME MESSAGES: The purpose of PTOA Segment #159 was to explore the second important PV Pressure - PV Flowrate relationship ... the PV Pressure ↔ Fluid Velocity Swap.
Simply stated, the PV Pressure ↔ Fluid Velocity Swap is the exchange of increased flow Velocity with decreased PV Pressure observed when a fluid flows through a restricted area.
Further downstream from the restriction, the PV Pressure increases ... or "swaps back" ... until it is restored to its original amount while the Velocity of the fluid correspondingly decreases ... or "swaps back" ... to its original amount.
The PV Pressure ↔ Fluid Velocity Swap ensures continuity of fluid flow even when the area of flow is decreased ... or increased.
Process technologies have harnessed the power of the PV Pressure ↔ Fluid Velocity Swap to:
- Detect and measure the PV (Volumetric) Flowrate as long as the flowing fluid can be characterized as Laminar Flow.
- Add Pressure to liquids (which are non-compressible) with centrifugal pumps.
Subject matter that supported full comprehension of the PV Pressure ↔ Fluid Velocity Swap included:
- The PV (Volumetric) Flowrate has two components, fluid Velocity and (Flow Through) Area.
- In a rigid pipe, the (Flow Through) Area is simply the circular area that is defined by the internal radius of the pipe.
- Laminar flow creates a "Velocity Profile" wherein the flow Velocity gradually increases "in radial layers" from the slowest flow velocity observed at the pipe's interior surface to the fastest flow velocity observed at the center of the pipe.
The two PV Pressure-PV Flowrate relationships featured in PTOA Segments #158 and #159 universally apply to freely flowing fluids AND fluids that flow through rigid plastic and metal pipes.
Without x-ray vision, the PV Pressure-PV Flowrate relationships cannot be observed at the processing plant but can be observed in freely flowing fluids.
©2017 PTOA Segment 0159
PTOA Process Variable Pressure Focus Study Area
PTOA PV Pressure Interrelationship with PV Flowrate
You need to login or register to bookmark/favorite this content.